Related Articles
Related Jobs
- How does “middling” work in betting?
- Are all points equal?
- Finding the value of a middle
Middling is a betting strategy that aims to exploit differential pricing between bookmakers or future line movements. It could be considered the betting market equivalent of statistical arbitrage, a trading strategy employed in financial markets. This article explains what middling is, and how you can profit from implementing this simple strategy.
How does “middling” work in betting?
We can use a practical example to understand how middling works. Consider a basketball game. Bookmaker A is quoting a game total of 204.5 and bookmaker B’s total is 200.5. To generate a middle, a bettor would bet ‘under 204.5’ at bookmaker A and ‘over 200.5’ at bookmaker B. A middle is created for totals of 201, 202, 203 and 204. If the total lands on any of these numbers, the bettor will win both bets. Notice that it’s impossible for the bettor to lose both bets. The worst case is losing one, and winning the other.
A middle can be generated on any line bet, be it a Handicap or Total. Determining if a potential middle is worth betting involves calculating the cost and estimating the chance of landing in the middle. Continuing the example above, assume that the odds offered on ‘under 204.5’ and ‘over 200.5’ are both 1.90, and a €100 bet is placed on each. Let’s consider the profit and loss for different game totals.
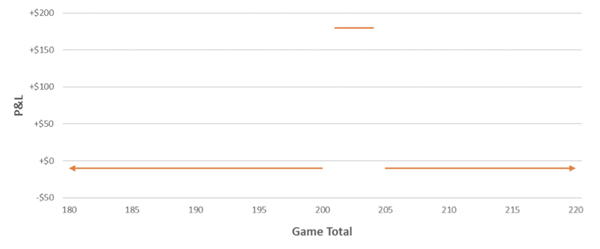
For the totals 201, 202, 203 and 204, the bettor will win both bets for a profit of €180 (+€90 from each bet). For all other totals, they will win one (+€90) and lose one (-€100) for a loss of -€10. When will betting this middle be a profitable strategy?
As with any betting strategy, the most important factors when middling are an accurate assessment of the likelihood of success, and being disciplined to ensure you pay the right price.
To answer this question let’s consider how often this middle would need to land for the bettor to break even. If the middle lands in the first game, the €180 profit could sustain the next 18 games of losses. Therefore, the break-even number of games is 19. So long as this middle lands in more than one in 19 games (5.3%), the strategy has positive expected value. In effect, betting this middle is equivalent to having a €10 bet at odds of 19.0.
What happens to the break-even percentage when the odds change? The chart below plots how often a middle needs to land for a bettor to break even at different odds. The assumption is that the odds are the same for each side of the middle.
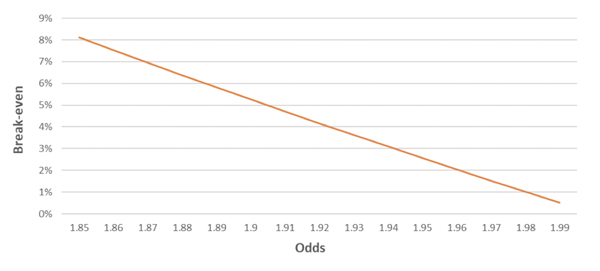
You may notice that the break-even percentage is equal to the bookmaker’s margin. For example, at odds of 1.95, a middle only needs to land 2.6% of the time to break-even, compared to 7.0% at odds of 1.87. To put it another way, betting at 1.95 compared to 1.87 is the difference between taking effective odds of 39.0 and 14.4 respectively, for a particular middle. The chart emphasises the importance of betting at the best possible odds when implementing a middling strategy.
Not all points are equal
When betting a middle, it’s important to understand the scoring system and patterns in the sport on which you’re betting. Some middles are more valuable than others. For example, in tennis, there are more potential score lines resulting in a differential of four games compared to three, and therefore a middle on the four-game Handicap will generally be more valuable (and thus more expensive) than a middle on the three-game Handicap.
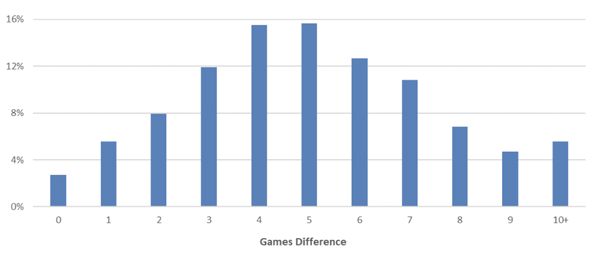
The chart below plots the distribution of the difference in games won for all ATP matches since 2007. A difference of four games occurs 30% more often than a difference of three.
Using Pinnacle’s odds to determine the value of a middle
A number of previous betting resources articles have shown Pinnacle’s odds to be highly efficient. Therefore, in the absence of our own model-derived probability for a certain middle landing, we can use Pinnacle’s odds to work out the likelihood using alternate lines. Consider the totals below for a random NBA match I pulled from Pinnacle’s site.
We could bet the 223 middle (over 222.5 and under 223.5), knowing from the chart above that we need this to land in 5.7% of games to break even (betting both sides at 1.892). Those that are familiar with basketball scoring will realise that this seems too high. Let’s instead use these odds to work out what Pinnacle believes the chances of the total landing 223 are.
To do this, first we need to remove the margin. I assume that the margin has been distributed in proportion to the implied probabilities. Pinnacle’s true probabilities are shown in the right column below.
Pinnacle’s probability of the total landing 223 is 2.8% (51.4% + 51.4% – 100%). From the break-even chart above, we would need odds greater than 1.95 each side for this to be a middle with a positive expected value.
Next let’s consider a tennis match. I chose a random ATP match and recorded game Handicap lines as quoted by Pinnacle. Using the same method as above, Pinnacle’s true probabilities are shown in the table below.
What are the odds saying about the probability of landing on the three middle: Player A winning three more games than Player B? The sum of the true probabilities for Player A -2.5 (58.6%) and Player B +3.5 (49.7%) is 108.2%, and therefore Pinnacle’s expectation of landing on this middle is 8.2%. Using this probability, and assuming one side is bet with Pinnacle, we can determine what odds we would need to take on the other side to generate a middle with positive expected value.
Pinnacle has Player A -2.5 odds of 1.628, implying a probability of 61.4%. What odds do we need on the other side of the middle (Player B +3.5)? An implied probability of 46.8% brings the sum to 108.2%, meaning we would require odds of 2.14 (1/0.468) or higher at another bookmaker to take this middle.
If we can bet at odds of 2.14 or above, we will have generated a middle with positive expected value. Similarly, assuming we bet with Pinnacle on the other side, Player B +3.5 at odds 1.943, we would require another bookmaker to offer odds above 1.76 on Player A -2.5.
Is middling worthwhile?
Middling is a great betting strategy to exploit differential pricing between bookmakers, especially when there is considerable disagreement in the market. It can also be used successfully when a future line movement can be predicted.
Middle bettors should be aware however, that variance will be high, as betting a middle is equivalent to backing a longshot. As with any betting strategy, the most important factors when middling are an accurate assessment of the likelihood of success, and being disciplined to ensure you pay the right price.

